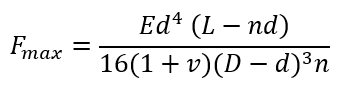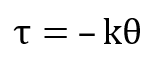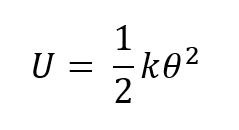Tension springs v torsion springs
Contents |
Introduction
Although sounding similar, tension and torsion springs are completely different. Tension springs are manufactured to operate with tension; as tightly wound coils, they stretch to a specific length as torque is applied to them. When in an unloaded position, the spring loops are touching with either a hook or a loop that is attached at one of the ends. The springs stretch when this attachment is directed with force. The springing action is caused when the components are pulled apart and the springs attempt to hold themselves together. After the force is stopped, they return to their original position.
Torsion springs are coiled springs, which work due to a twisting or torsion rotational motion. This allows for an object to store mechanical energy as it is twisted, exerting force in the opposite direction depending on the angle that it is twisted. A helical looped design is the most common design for torsion springs, which consists of a coiled metal wire that is twisted tighter and tighter by sideways forces that are applied on either a left-hand or a right-hand helix.
Tension Springs
Most springs follow Hooke’s Law of elasticity, which states that the extension of an elastic rod is linearly proportional to the force, or torque, used to stretch it. The law is held approximately when the deformation is considered to be small in comparison to the overall length of the rod. The equation used to represent the force of a fully compressed spring is the following:
In which:
E – is Young’s modulus
d – is the spring wire diameter
L – is the free length of the spring
n – is the number of active windings
v – is Poisson’s ratio
D – is the spring’s outer diameter
Torsion Springs
Torsion springs work by either twisting or torsion, in which they store mechanical energy when twisted. When they are twisted, the springs exert torque in the opposite direction in a proportional amount to the angle that they are twisted.
These springs obey Hooke’s Law, as long as not twisted beyond the elastic limit:
In which τ represents the exerted torque by the spring in newton-metres, k is a constant of newton-metres units, and θ represents the angle of twist from the equilibrium position in radians.
In joules, U, the energy stored in a torsion spring is represented by the following equation:
Uses of Tension Springs
Uses of Torsion Springs
--European Springs and Pressings Ltd 09:44, 25 Sep 2017 (BST)
Related articles on Designing Buildings Wiki
- Compression springs.
- E-spring.
- Flat springs.
- Key qualities of springs.
- Spring materials.
- The importance of gas springs.
- Using springs in construction to prevent disaster.
- The Difference Between Tension and Torsion Springs
- The Multiple Uses of Compression Springs
- The properties of tension springs.
- Using Springs in Construction to Prevent Disaster
- Torsion Springs and Their Benefits
Featured articles and news
Latest Build UK Building Safety Regime explainer published
Key elements in one short, now updated document.
UKGBC launch the UK Climate Resilience Roadmap
First guidance of its kind on direct climate impacts for the built environment and how it can adapt.
CLC Health, Safety and Wellbeing Strategy 2025
Launched by the Minister for Industry to look at fatalities on site, improving mental health and other issues.
One of the most impressive Victorian architects. Book review.
Common Assessment Standard now with building safety
New CAS update now includes mandatory building safety questions.
RTPI leader to become new CIOB Chief Executive Officer
Dr Victoria Hills MRTPI, FICE to take over after Caroline Gumble’s departure.
Social and affordable housing, a long term plan for delivery
The “Delivering a Decade of Renewal for Social and Affordable Housing” strategy sets out future path.
A change to adoptive architecture
Effects of global weather warming on architectural detailing, material choice and human interaction.
The proposed publicly owned and backed subsidiary of Homes England, to facilitate new homes.
How big is the problem and what can we do to mitigate the effects?
Overheating guidance and tools for building designers
A number of cool guides to help with the heat.
The UK's Modern Industrial Strategy: A 10 year plan
Previous consultation criticism, current key elements and general support with some persisting reservations.
Building Safety Regulator reforms
New roles, new staff and a new fast track service pave the way for a single construction regulator.
Architectural Technologist CPDs and Communications
CIAT CPD… and how you can do it!
Cooling centres and cool spaces
Managing extreme heat in cities by directing the public to places for heat stress relief and water sources.
Winter gardens: A brief history and warm variations
Extending the season with glass in different forms and terms.
Restoring Great Yarmouth's Winter Gardens
Transforming one of the least sustainable constructions imaginable.