Net Present Value
Contents |
[edit] Introduction
NRM3: Order of cost estimating and cost planning for building maintenance works, defines 'present value' as '...the cost or benefit in the future discounted back to some base date, usually the present day, at a given compound interest rate'.
The term ‘Net Present Value’ (NPV) represents the difference between the present value of cash inflows and the present value of cash outflows for an investment. It is used when considering capital investments to assess profitability.
For an investment to be worthwhile it has to yield a positive NPV, meaning that profit will be generated over time as a result of the investment. A negative NPV indicates that the investment is likely to lose money. Like any other business investment, property development will aim to yield a positive NPV that is greater than would have been achieved if capital was invested elsewhere.
NRM3 suggests that:
| NPV is a standard measure in LCC (life cycle cost) analyses, used to determine and compare the cost effectiveness of proposed solutions. It can be applied across the full range of construction investments, covering whole investment programmes, assets, systems, components and operating and maintenance models.The costs and revenues/benefits to be included in each analysis are defined according to its objectives. For example, revenues from recycling of materials or from surplus energy generation are typically included in LCC analysis of alternative sustainability options. |
[edit] Formula
The formula for calculating NPV is as follows:
Where:
- Ct = net cash inflow during the period ‘t’
- Co = total initial investment costs
- r = discount rate
- t = number of time periods
[edit] Example
A construction project has initial costs of £1.7m. It is expected to generate the following cash inflow:
- End of year 1 = £120,000.
- End of year 2 = £250,000.
- End of year 3 = £550,000.
- End of year 4 = £1.3m.
Without applying discounts for depreciation, the NPV of the project is:
NPV = £2.22m – £1.7m
NPV = £520,000
Without discounting there is sufficient economic justification for the project to go ahead.
Discounting is a way of comparing the value of costs and benefits over different time periods relative to their present values. Money is worth less in the future than it is in the present because of its reduced capacity for generating a return, such as interest, and because of inflation. Discounting is a means of assessing how much less an amount is worth in the future than it is now.
As property development and construction generally face significant costs over long periods of time, they are particularly susceptible to discount rate sensitivity.
With a 5% discount rate applied to the example project, the NPV becomes:
(Y1) £114,285.70 + (Y2) £226,757.37 + (Y3) £475,110.68 + (Y4) £1,069,513.22
NPV = £1,885,666.97
NPV = 1,885,666.97 – £1.7m
NPV = £185,666.97
So there is still economic justification for the project to go ahead. However, if the discount rate is increased to 10% the NPV is:
(Y1) £109,090.91 + (Y2) £206,611.57 + (Y3) £413,223.14 + (Y4) £887,917.49
NPV = £1,616,843.11
NPV = £1,616,843.11 – £1.7m
NPV = -£83,156.89
In this scenario there appears not to be economic justification for the project to go ahead.
Understanding NPV can help assess whether to proceed with a project, how profitability compares with alternative investments, or may help negotiate down prices.
[edit] Drawbacks of using NPV
As an analysis tool, NPV has a number of drawbacks:
- Estimated cash flows seldom match those experienced in practice.
- Given the incremental cost of capital required to fund a project, a simple discount rate may not adequately represent the situation.
- Adjustments to take account of risks will only be very rough estimate estimates.
- NPV analysis only considers the circumstances of a specific investment.
[edit] Net present social value
The Green Book, Central Government Guidance On Appraisal And Evaluation, Published by HM Treasury in 2020, defines Net Present Value (NPV) as; ‘a generic term for the sum of a stream of future values (that are already in real prices) that have been discounted (in the Green Book by the social time preference rate) to bring them to today’s value.’
It defines Net Present Social Value (NPSV) or Net Present Public Value (NPPV): '...the present value of a stream of future costs and benefits to UK society (that are already in real prices) and that have been discounted over the life of a proposal by the appropriate Green Book social time preference rate.'
[edit] Other definitions
Life Cycle Costing (BG 67/2016), written by David Churcher and Peter Tse and published by BSRIA in March 2016, defines present value as: ‘The value of a future cost or benefit at the present time. A future value is discounted by applying the discount rate and knowing how many years (or time periods) into the future it occurs.’
[edit] Related articles on Designing Buildings
- Base year.
- Budget.
- Business plan.
- Capex.
- Capital allowances.
- Capital costs for construction projects.
- Cash flow.
- Compound Annual Growth Rate (CAGR).
- Cost performance index (CPI).
- Cost-benefit analysis in construction.
- Development appraisal.
- Discounting.
- Discount rate.
- Discounted cash flow.
- Gross value added (GVA).
- Internal rate of return for property development.
- Investment.
- Net benefits.
- Net savings.
- Life cycle assessment.
- Life Cycle Costing BG67 2016.
- Time value of money.
- Whole life costs.
- Yield.
[edit] External references
Featured articles and news
Investors in People: CIOB achieves gold
Reflecting a commitment to employees and members.
Scratching beneath the surface; a guide to selection.
ECA 2024 Apprentice of the Year Award
Entries open for submission until May 31.
UK gov apprenticeship funding from April 2024
Brief summary the policy paper updated in March.
For the World Autism Awareness Month of April.
70+ experts appointed to public sector fire safety framework
The Fire Safety (FS2) Framework from LHC Procurement.
Project and programme management codes of practice
CIOB publications for built environment professionals.
The ECA Industry Awards 2024 now open !
Recognising the best in the electrotechnical industry.
Sustainable development concepts decade by decade.
The regenerative structural engineer
A call for design that will repair the natural world.
Buildings that mimic the restorative aspects found in nature.
CIAT publishes Principal Designer Competency Framework
For those considering applying for registration as a PD.
BSRIA Building Reg's guidance: The second staircase
An overview focusing on aspects which most affect the building services industry.
Design codes and pattern books
Harmonious proportions and golden sections.
Introducing or next Guest Editor Arun Baybars
Practising architect and design panel review member.
Quick summary by size, shape, test, material, use or bonding..







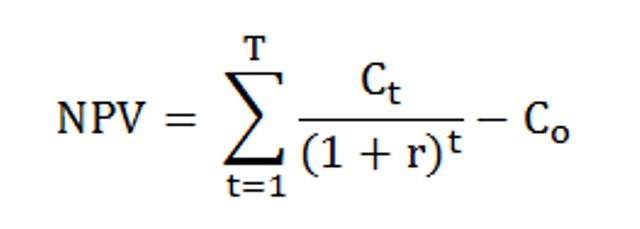
















Comments