Compound Annual Growth Rate (CAGR)
Compound annual growth rate (CAGR) is a measure of the mean annual growth rate of an investment over a specified time period. It measures the growth rate effecting the value of the initial investment to that of the end-of-period investment, with the assumption that over that time period the investment has been compounding.
While CAGR isn’t a true return rate, it is a representational figure used to understand an investment’s returns and is considered a better measure of return over time. The effects of compounding are ignored by the average annual return figures, which can overestimate an investment’s growth. By contrast, CAGR uses a geometric average to represent the consistent rate at which, if compounding had occurred at the same annual rate, the investment would have grown.
The formula for CAGR is:
CAGR = (EV / BV)^(1 / n) - 1
where:
- EV = Investment's end-of-period value
- BV = Investment's beginning value
- n = Number of periods (months, years, etc.)
As an example, if an investment of £2,000 is made for six years, with year-end values of the investment as follows:
- Year 1: £1,500
- Year 2: £2,000
- Year 3: £6,000
- Year 4: £8,000
- Year 5: £10,000
- Year 6: £12,000
The CAGR is: (12,000 / 2,000)^(1/6) – 1 = 0.348 = 34.8%
As it is a simple metric, CAGR is also flexible and can be used in a variety of ways, for example, comparing investments of different types, or tracking the performance of various measures alongside one another.
However, it should be used with other metrics to give a representative overall picture as it does have some limitations. For instance, it ignores volatility and implies that growth over the time period was steady, whereas in reality growth can be higher or lower from one year to the next. In addition, CAGR does not predict that the investment will continue to grow at the same rate, as it is only a historical metric. Many other factors might affect the rate of growth in future years.
[edit] Find out more
[edit] Related articles on Designing Buildings Wiki
- Accounting.
- Base year.
- Cash flow.
- Cost engineering.
- Cost performance index (CPI).
- Cost-benefit analysis in construction.
- Discounting.
- Gross value added (GVA).
- Internal rate of return.
- Net Present Value.
- Profitability.
- Residual value.
- Turnover.
- Yield.
[edit] External resources
- Investopedia - CAGR
Featured articles and news
RTPI leader to become new CIOB Chief Executive Officer
Dr Victoria Hills MRTPI, FICE to take over after Caroline Gumble’s departure.
Social and affordable housing, a long term plan for delivery
The “Delivering a Decade of Renewal for Social and Affordable Housing” strategy sets out future path.
A change to adoptive architecture
Effects of global weather warming on architectural detailing, material choice and human interaction.
The proposed publicly owned and backed subsidiary of Homes England, to facilitate new homes.
How big is the problem and what can we do to mitigate the effects?
Overheating guidance and tools for building designers
A number of cool guides to help with the heat.
The UK's Modern Industrial Strategy: A 10 year plan
Previous consultation criticism, current key elements and general support with some persisting reservations.
Building Safety Regulator reforms
New roles, new staff and a new fast track service pave the way for a single construction regulator.
Architectural Technologist CPDs and Communications
CIAT CPD… and how you can do it!
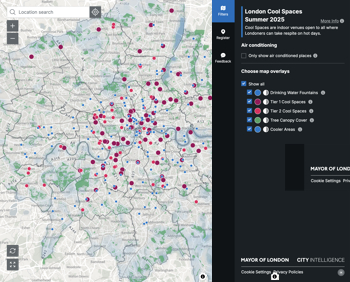
Cooling centres and cool spaces
Managing extreme heat in cities by directing the public to places for heat stress relief and water sources.
Winter gardens: A brief history and warm variations
Extending the season with glass in different forms and terms.
Restoring Great Yarmouth's Winter Gardens
Transforming one of the least sustainable constructions imaginable.
Construction Skills Mission Board launch sector drive
Newly formed government and industry collaboration set strategy for recruiting an additional 100,000 construction workers a year.
New Architects Code comes into effect in September 2025
ARB Architects Code of Conduct and Practice available with ongoing consultation regarding guidance.
Welsh Skills Body (Medr) launches ambitious plan
The new skills body brings together funding and regulation of tertiary education and research for the devolved nation.
Paul Gandy FCIOB announced as next CIOB President
Former Tilbury Douglas CEO takes helm.
UK Infrastructure: A 10 Year Strategy. In brief with reactions
With the National Infrastructure and Service Transformation Authority (NISTA).